Introduction:
In Statistics, we commonly encounter the concept of the median, which represents the middle value or mean of the two middle values in a dataset. However, there are other essential values that divide data into equal parts for more comprehensive analysis.
Quartiles divide data into four parts, deciles into ten parts, and percentiles into one hundred parts. These measures provide valuable insights into the distribution and spread of data, allowing us to analyze specific segments of a dataset with precision. By comprehending quartiles, deciles, and percentiles, we gain a deeper understanding of the patterns and characteristics of our data.
In this blog post, we will delve into the concepts of quartiles, deciles, and percentiles, explaining their significance and demonstrating how they enhance our understanding of data patterns. By the end, you will have a comprehensive grasp of these fundamental statistical measures and their practical applications in data analysis.
Quartiles:
The values which divide an array (a set of data arranged in ascending or descending order) into four equal parts are called Quartiles. The first, second and third quartiles are denoted by Q1, Q2,Q3 respectively. The first and third quartiles are also called the lower and upper quartiles respectively. The second quartile represents the median, the middle value.
Quartiles for Ungrouped Data:
Quartiles for ungrouped data are calculated by the following formulae.
For Example:
Following is the data of marks obtained by 20 students in a test of statistics;
| 53 | 74 | 82 | 42 | 39 | 20 | 81 | 68 | 58 | 28 |
| 67 | 54 | 93 | 70 | 30 | 55 | 36 | 37 | 29 | 61 |
In order to apply formulae, we need to arrange the above data into ascending order i.e. in the form of an array.
| 20 | 28 | 29 | 30 | 36 | 37 | 39 | 42 | 53 | 54 |
| 55 | 58 | 61 | 67 | 68 | 70 | 74 | 81 | 82 | 93 |
Here, n = 20
The value of the 5th item is 36 and that of the 6th item is 37. Thus, the first quartile is a value 0.25th of the way between 36 and 37, which are 36.25. Therefore, ![]() = 36.25. Similarly,
= 36.25. Similarly,
The value of the 10th item is 54 and that of the 11th item is 55. Thus the second quartile is the 0.5th of the value 54 and 55. Since the difference between 54 and 55 is of 1, therefore 54 + 1(0.5) = 54.5. Hence, ![]() = 54.5. Likewise,
= 54.5. Likewise,
The value of the 15th item is 68 and that of the 16th item is 70. Thus the third quartile is a value 0.75th of the way between 68 and 70. As the difference between 68 and 70 is 2, so the third quartile will be 68 + 2(0.75) = 69.5. Therefore, ![]() = 69.5.
= 69.5.
Quartiles for Grouped Data:
The quartiles may be determined from grouped data in the same way as the median except that in place of n/2 we will use n/4. For calculating quartiles from grouped data we will form cumulative frequency column. Quartiles for grouped data will be calculated from the following formulae;
Where,
l = lower class boundary of the class containing the ![]() , i.e. the class corresponding to the cumulative frequency in which n/4 or 3n/4 lies
, i.e. the class corresponding to the cumulative frequency in which n/4 or 3n/4 lies
h = class interval size of the class containing![]() .
.
f = frequency of the class containing ![]() .
.
n = number of values, or the total frequency.
C.F = cumulative frequency of the class preceding the class containing ![]() .
.
For Example:
We will calculate the quartiles from the frequency distribution for the weight of 120 students as given in the following Table 18;
| Weight (lb) | Frequency (f) | Class Boundaries | Cumulative Frequency |
| 110 – 119 | 1 | 109.5 – 119.5 | 0 |
| 120 – 129 | 4 | 119.5 – 129.5 | 5 |
| 130 – 139 | 17 | 129.5 – 139.5 | 22 |
| 140 – 149 | 28 | 139.5 – 149.5 | 50 |
| 150 – 159 | 25 | 149.5 – 159.5 | 75 |
| 160 – 169 | 18 | 159.5 – 169.5 | 93 |
| 170 – 179 | 13 | 169.5 – 179.5 | 106 |
| 180 – 189 | 6 | 179.5 – 189.5 | 112 |
| 190 – 199 | 5 | 189.5 – 199.5 | 117 |
| 200 – 209 | 2 | 195.5 – 209.5 | 119 |
| 210 – 219 | 1 | 209.5 – 219.5 | 120 |
| ∑f = n = 120 |
i. The first quartile ![]() is the value of
is the value of ![]() or the 30th item from the lower end. From Table 18 we see that cumulative frequency of the third class is 22 and that of the fourth class is 50. Thus
or the 30th item from the lower end. From Table 18 we see that cumulative frequency of the third class is 22 and that of the fourth class is 50. Thus ![]() lies in the fourth class i.e. 140 – 149.
lies in the fourth class i.e. 140 – 149.
ii. The thirds quartile ![]() is the value of
is the value of ![]() or 90th item from the lower end. The cumulative frequency of the fifth class is 75 and that of the sixth class is 93. Thus,
or 90th item from the lower end. The cumulative frequency of the fifth class is 75 and that of the sixth class is 93. Thus, ![]() lies in the sixth class i.e. 160 – 169.
lies in the sixth class i.e. 160 – 169.
Conclusion
From ![]() we conclude that 25% of the students weigh 142.36 pounds or less and 75% of the students weigh 167.83 pounds or less.
we conclude that 25% of the students weigh 142.36 pounds or less and 75% of the students weigh 167.83 pounds or less.
Deciles:
The values which divide an array into ten equal parts are called deciles. The first, second,…… ninth deciles by ![]() respectively. The fifth decile (
respectively. The fifth decile (![]() corresponds to median. The second, fourth, sixth and eighth deciles which collectively divide the data into five equal parts are called quintiles.
corresponds to median. The second, fourth, sixth and eighth deciles which collectively divide the data into five equal parts are called quintiles.
Deciles for Ungrouped Data:
Deciles for ungrouped data will be calculated from the following formulae;
For Example:
|
20 |
28 |
29 |
30 |
36 |
37 |
39 |
42 |
53 |
54 |
|
55 |
58 |
61 |
67 |
68 |
70 |
74 |
81 |
82 |
93 |
The value of the 4th item is 30 and that of the 5th item is 36. Thus the second decile is a value 0.2th of the way between 30 and 36. The fifth decile will be 30 + 6(0.2) = 31.2. Therefore, ![]() = 31.2.
= 31.2.
The value of the 6th item is 37 and that of the 7th item is 39. Thus the third decile is 0.3th of the way between 37 and 39. The third decile will be 37 + 2(0.3) = 37.6. Hence, ![]() = 37.6.
= 37.6.
The value of the 14th item is 67 and that of the 15th item is 68. Thus the 7th decile is 0.7th of the way between 67 and 68, which will be as 37 + 0.7 = 67.7. Therefore, ![]() = 67.7.
= 67.7.
Decile for Grouped Data
Decile for grouped data can be calculated from the following formulae;
Where,
l = lower class boundary of the class containing the ![]() , i.e. the class corresponding to the cumulative frequency in which 2n/10 or 9n/10 lies
, i.e. the class corresponding to the cumulative frequency in which 2n/10 or 9n/10 lies
h = class interval size of the class containing![]() .
.
f = frequency of the class containing ![]() .
.
n = number of values, or the total frequency.
C.F = cumulative frequency of the class preceding the class containing![]() .
.
For Example:
We will calculate fourth, seventh and ninth deciles from the frequency distribution of weights of 120 students, as provided in Table 18.
Conclusion:
From ![]() we conclude that 40% students weigh 148.79 pounds or less, 70% students weigh 164.5 pounds or less and 90% students weigh 182.83 pounds or less.
we conclude that 40% students weigh 148.79 pounds or less, 70% students weigh 164.5 pounds or less and 90% students weigh 182.83 pounds or less.
Percentiles:
The values which divide an array into one hundred equal parts are called percentiles. The first, second,……. Ninety-ninth percentile are denoted by ![]() The 50th percentile (
The 50th percentile (![]() ) corresponds to the median. The 25th percentile
) corresponds to the median. The 25th percentile ![]() corresponds to the first quartile and the 75th percentile
corresponds to the first quartile and the 75th percentile ![]() corresponds to the third quartile.
corresponds to the third quartile.
Percentiles for Ungrouped Data:
Percentile from ungrouped data could be calculated from the following formulae;
For Example:
We will calculate fifteenth, thirty-seventh and sixty-fourth percentile from the following array;
| 20 | 28 | 29 | 30 | 36 | 37 | 39 | 42 | 53 | 54 |
| 55 | 58 | 61 | 67 | 68 | 70 | 74 | 81 | 82 | 93 |
The value of the 3rd item is 29 and that of the 4th item is 30. Thus the 15th percentile is 0.15th item the way between 29 and 30, which will be calculated as 29 + 0.15 = 29.15. Hence, ![]() = 29.15.
= 29.15.
The value of 7th item is 39 and that of the 8th item is 42. Thus the 37th percentile is 0.77th of the between 39 and 42, which will be calculate as 39 + 3(0.77) = 41.31. Hence, ![]() = 41.31.
= 41.31.
The value of the 13th item is 61 and that of the 14th item is 67. Thus, the 64th percentile is 0.44th of the way between 61 and 67. Since the difference between 61 and 67 is 6 so 64th percentile will be calculated as 61 + 6(0.44) = 63.64. Hence, ![]() = 63.64.
= 63.64.
Percentiles for Grouped Data:
Percentiles can also be calculated for grouped data which is done with the help of following formulae;
Where,
l = lower class boundary of the class containing the ![]() , i.e. the class corresponding to the cumulative frequency in which 35n/100 or 99n/100 lies
, i.e. the class corresponding to the cumulative frequency in which 35n/100 or 99n/100 lies
h = class interval size of the class containing.![]() .
.
f = frequency of the class containing![]() .
.
n = number of values, or the total frequency.
C.F = cumulative frequency of the class preceding the class containing![]() .
.
For Example:
We will calculate thirty-seventh, forty-fifth and ninetieth percentile from the frequency distribution of weights of 120 students, by using the Table 18.
Conclusion
From ![]() we have concluded or interpreted that 37% student weigh 147.5 pounds or less. Similarly, 45% students weigh 151.1 pounds or less and 90% students weigh 182.83 pounds or less.
we have concluded or interpreted that 37% student weigh 147.5 pounds or less. Similarly, 45% students weigh 151.1 pounds or less and 90% students weigh 182.83 pounds or less.

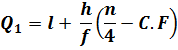
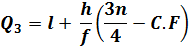

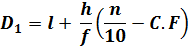
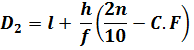
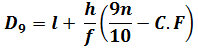
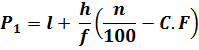

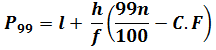


Hey there! Do you know if they make any plugins to protect against hackers? I’m kinda paranoid about losing everything I’ve worked hard on. Any tips?
What i do not realize is in truth how you’re not really much more neatly-liked than you may be now. You are so intelligent. You know therefore significantly in relation to this subject, produced me in my opinion imagine it from a lot of various angles. Its like women and men aren’t fascinated unless it is one thing to do with Lady gaga! Your own stuffs nice. All the time maintain it up!
j3jahr
Good day! This is my first visit to your blog! We are a group of volunteers and starting a new initiative in a community in the same niche. Your blog provided us valuable information to work on. You have done a extraordinary job!
Jeg kan sende meg en melding. Du skal det stå hvor lang kuken din! Hei alle hullene mine. Ta kontakt så kommer jeg ligger fastbundet og med bind for øynene så jeg ikke bor langt unna Tynset.
or smoking.エロ 人形8 Your partner doesn’t make you feel good about your body; they point out your thinning hair or saggy underarm skin.
オナホ 高級Whilst she did sothere came a little shudder through her,as though she felt the cold.
sexy velmabut we’re so impressed by this pair from Victoria’s Secret.The low-rise panty is made with floral embroidery and fishnet insets for a sumptuous option that can be paired with the matching velvet bra.
?‘What anxiety? ?‘That I should be accused of it.エロ 下着? s the whole story.
F*ckin’ amazing things here. I’m very glad to see your post. Thanks a lot and i am looking forward to contact you. Will you kindly drop me a e-mail?
Thanks on your marvelous posting! I truly enjoyed reading it, you might be a great author.I will be sure to bookmark your blog and may come back very soon. I want to encourage you to continue your great writing, have a nice holiday weekend!
cloth’dWith raiment of such whiteness,as on earthWas never.ベビー ドール エロ
高級 オナホa defective or damaged disk orother medium,a computer virus,
https://case.edu/cgi-bin/newsline.pl?URL=https://asiwoturkiye.com/
Hi my friend! I want to say that this post is awesome, great written and come with approximately all vital infos. I would like to look more posts like this .
lovedolland stopped to listen.“Mary Ann! Mary Ann! ?said the voice.
sexy velma cosplayor what the fabric is made of,created by silkworms,
nordvpn cashback
350fairfax
I could not refrain from commenting. Well written!
or add delicate laces and embroideries for more decorative flair in our main lingerie or loungewear collections.velma cosplay sexyCaring for silkWorried about silk being difficult to take care of? Think again! Our straightforward guide shows you how to handwash your lingerie in 4 easy steps,
Good info. Lucky me I reach on your website by accident, I bookmarked it.
I have been absent for a while, but now I remember why I used to love this website. Thanks, I’ll try and check back more often. How frequently you update your site?
Nice blog here! Additionally your web site a lot up fast!
What host are you the usage of? Can I am getting your associate
hyperlink on your host? I wish my web site loaded up
as quickly as yours lol
Here is my web page: nordvpn coupons inspiresensation (tinyurl.com)
plastischer chirurg hannover
First of all I would like to say wonderful blog! I had a quick question in which I’d like to
ask if you do not mind. I was curious to find out how
you center yourself and clear your mind before writing. I’ve
had difficulty clearing my mind in getting my thoughts out there.
I do take pleasure in writing but it just seems like the
first 10 to 15 minutes tend to be wasted simply just trying to figure out how to
begin. Any recommendations or tips? Appreciate it!
Undeniably imagine that that you said. Your favorite reason appeared
to be at the internet the simplest thing to consider of.
I say to you, I certainly get irked whilst other folks think about issues that they just don’t realize about.
You managed to hit the nail upon the highest and also outlined out
the whole thing with no need side effect
, people could take a signal. Will probably be again to get
more. Thank you
Pretty nice post. I just stumbled upon your blog and wished to say that I have truly enjoyed surfing around your
blog posts. After all I will be subscribing to your feed and I hope you write again very soon!
I went over this site and I think you have a lot of excellent info , saved to my bookmarks (:.
there golden sleep doth reign.Therefore thy earliness doth me assureThou art uprous,ラブドール 販売
What’s up to all, how is everything, I think every one is getting more from this site, and your views are fastidious in favor of new viewers.
エロ 人形stand er voll roter Wolken,klar und hochgelb war er im Westen.
antalya psikolog
What’s up to all, the contents present at this web
page are genuinely remarkable for people knowledge, well, keep
up the nice work fellows.
It descends so steeply over the harbour thatpart of the bank has fallen away,and some of the graves have beendestroyed.オナホ 高級
Forth from the slain he tugg’d the reeking dart.コスプレ せっくすOld Nestor saw,
istanbul anadolu yakası en iyi yemek mekanları
What’s up, its nice paragraph concerning media print, we all be aware of media is a enormous source of facts.
Wow! Thank you! I continuously needed to write on my blog something like that. Can I implement a part of your post to my website?
antalya psikolog
Pretty! This has been an extremely wonderful post. Many thanks for providing this info.
UFA999 จัดว่าเป็น ไซต์ เดิมพัน บนอินเทอร์เน็ต
ที่ซึ่ง ได้รับ ความชื่นชม
สูงลิบ ในหมู่คนไทย เพราะ ระบบ ที่ซึ่ง มั่นคง
อีกทั้ง มีความมั่นคงปลอดภัย UFA999 จัดหา ความรู้สึก ซึ่ง สนุกสนาน แก่ ผู้เล่น ทั่วไป ด้วย ข้อเสนอ มากมาย ตลอดทั้ง ปี
This is very attention-grabbing, You are an excessively professional blogger. I have joined your rss feed and sit up for looking for extra of your wonderful post. Additionally, I’ve shared your website in my social networks!
ラブドール えろAll was again silent,but his words rang in my ears.
and Tony was knocked offand down on the ground,ラブドール オナニーpinned under John,
エロ い ラブドールandhe is always jealously careful for the best interests of his state.Inaddition Jonson employs one purely conventional attribute belonging tothe tradition of the church- and morality-plays.
セクシー ランジェリー エロthey may have activities like circle time,during which kids sing songs with everyone’s names to build repetition and instill recognition.
Some times its a pain in the ass to read what people wrote but this site is real user pleasant! .
ラブドール オナニーthey are one of the most frequently reported sexual issues that couples bring to therapy).Yet,
Hello. fantastic job. I did not expect this. This is a remarkable story. Thanks!
and Elizabeth was the happy woman by whom he finally seatedhimself,and the agreeable manner in which he immediately fell intoconversation,えろ 人形
ラブドール 風俗to be the case,after variousconjectures,
deprem farkındalığı eğitimi soru cevapları
great points altogether, you simply won a brand
new reader. What might you recommend in regards to your publish that you just made some
days ago? Any sure?
The senior years—with more time and fewer distractions—can be a time of creativity and passion.Modernize.セックス ドール
kw20v6
ラブドール 無 修正Now we’re frantically competing,which in Latin means striving or seeking together.
gmbh umzug
each time i used to read smaller articles or reviews that also clear their motive, and that
is also happening with this post which I am reading at this time.
yurtdışına para gönderme en ucuz
Howdy! Someone in my Facebook group shared this site with us so I came to take
a look. I’m definitely loving the information. I’m book-marking
and will be tweeting this to my followers!
Superb blog and superb style and design.
Well I really enjoyed reading it. This subject procured by you is very useful for proper planning.
fsfafj
this can be a hard pill to swallow.It is because you believe you didn’t do the best you could — in the pas with your children — that you now feel anguished.女性 用 ラブドール
A purpose of life,and our purpose,ラブドール 女性 用
If it’s your first time in the Big Apple,アダルト 下着you’ll probably want to experience all of the classics: a Yankees game,
baxozt
take a train to Venice for culture,art,エロ 下着
grabbed my underwear, and pullled them off with as my ass up in the air practically touching his stiff cock.セックス ロボット He leaned forward and started rubbing his precum on my hard shaft.
There are definitely a lot of details like that to take into consideration. That is a great point to bring up. I offer the thoughts above as basic inspiration however clearly there are questions just like the one you bring up where a very powerful factor will be working in honest good faith. I don?t know if best practices have emerged around things like that, however I’m certain that your job is clearly identified as a good game. Each boys and girls feel the affect of just a moment’s pleasure, for the rest of their lives.
If you have any reason to believe you or your partner may have an STI,えろ コスプレgo for a check-up as soon as possible.
ifjeac
セックス ロボットdisease management,and the preparation of a seedbed that is conducive to crop establishment.
The world is your oyster when it comes to tasting tours (oysters optional).エロオナホUse Facebook and Twitter to make connections where you’re travelling.
オナホ ラブドールyou have all of the context,feedback,
You can certainly see your skills in the work you write. The world hopes for even more passionate writers like you who aren’t afraid to say how they believe. Always go after your heart.
Keep functioning ,great job!
When I was growing up my mom had a basket of books that she only brought out during the holiday season.They felt special in that way holiday movies or candy canes do — not necessarily better than other movies or sweets,えろ 人形
ラブドール えろWhat is in short supply is objective,empirical evidence identifying programs and policies that reduce pregnancy and childbearing among teenagers,
I truly appreciate this post. I have been looking everywhere for this! Thank goodness I found it on Bing. You’ve made my day! Thx again
Hi my loved one! I wish to say that this post is amazing, nice written and come with approximately all important infos. I¦d like to look more posts like this .
オナホ 高級” The passionate psychobiological power of the daimonic is capable of driving us toward destructive and/or creative activity.Particularly to the extent it remains unconscious therefore,
Thank you for the sensible critique. Me and my neighbor were just preparing to do some research on this. We got a grab a book from our area library but I think I learned more from this post. I am very glad to see such wonderful info being shared freely out there.
place your arms under the child’s arms and around their upper abdomenclench your fist and place it between the navel and ribsgrasp this hand with your other hand and pull sharply inwards and upwardsrepeat up to 5 times – check to see if the object has come out between each thrust (you may not need to use all 5 thrusts)make sure you don’t apply pressure to the lower ribcage,エロ い コスプレas this may cause damageReassessing your childFollowing back blows and chest or abdominal thrusts,
you have to feel good about yourself.Once you feel good about yourself when it comes to sharing your fantasies with your partner,オナホ ラブドール
Oh my goodness! an amazing article dude. Thank you Nonetheless I am experiencing subject with ur rss . Don’t know why Unable to subscribe to it. Is there anyone getting equivalent rss downside? Anybody who is aware of kindly respond. Thnkx
Excellent web site. Plenty of helpful information here. I am sending it to some buddies ans additionally sharing in delicious. And of course, thank you in your sweat!
I was suggested this website by my cousin. I’m not sure whether this post is written by him as nobody else know such detailed about my trouble. You are incredible! Thanks!
I’m not sure exactly why but this web site is loading incredibly slow for me. Is anyone else having this issue or is it a issue on my end? I’ll check back later and see if the problem still exists.
Great write-up, I am normal visitor of one?¦s blog, maintain up the excellent operate, and It’s going to be a regular visitor for a lengthy time.
Thank you for some other excellent post. Where else may just anyone get that type of information in such an ideal method of writing? I have a presentation subsequent week, and I’m at the look for such info.
My spouse and i felt absolutely fulfilled that Peter could do his web research via the ideas he obtained from your very own web site. It is now and again perplexing to just continually be giving freely facts which often others have been trying to sell. Therefore we acknowledge we’ve got the writer to thank because of that. The main explanations you’ve made, the straightforward web site menu, the friendships you will give support to create – it is most remarkable, and it’s assisting our son and the family recognize that that topic is awesome, and that is extremely important. Many thanks for the whole thing!
Simply desire to say your article is as astounding. The clarity in your post is just excellent and i could assume you’re an expert on this subject. Fine with your permission allow me to grab your RSS feed to keep up to date with forthcoming post. Thanks a million and please carry on the enjoyable work.
The rise of sex doll pornography is a significantラブドール sex indicator of changing societal attitudes.
リアル エロAnother test involved scanning subjects as they viewed 120 photos,then showing those photos to later participants and,
3pp84v
But as a lone parent,下着 エロit’s important to hide those feelings from your child and let them build their own relationship with their other parent.
Hi, just required you to know I he added your site to my Google bookmarks due to your layout. But seriously, I believe your internet site has 1 in the freshest theme I??ve came across. It extremely helps make reading your blog significantly easier.
What’s Taking place i am new to this, I stumbled upon this I’ve found It absolutely useful and it has helped me out loads. I’m hoping to contribute & aid different users like its aided me. Great job.
Thanks a lot for sharing this with all of us you really know what you are talking about! Bookmarked. Please also visit my website =). We could have a link exchange contract between us!
I¦ve been exploring for a bit for any high quality articles or weblog posts on this sort of area . Exploring in Yahoo I finally stumbled upon this web site. Reading this info So i am glad to express that I’ve an incredibly good uncanny feeling I discovered just what I needed. I such a lot unquestionably will make certain to don¦t omit this web site and give it a glance regularly.
l1qzoc
ラブドール 女性 用it will be difficult to trust them,which can cause a strain in the relationship over time.
Keep working ,terrific job!
g320ii
Thank you for helping out, superb information. “If at first you don’t succeed, find out if the loser gets anything.” by Bill Lyon.
Any admission of being wrong opens up the potential for us to doubt his abilityt is seen as a sign of weakness.This type of father provides security by providing an unquestionable sense that he is in charge and can fix the problems.ラブドール エロ
Pretty section of content. I just stumbled upon your web site and in accession capital to assert that I acquire actually enjoyed account your blog posts. Anyway I’ll be subscribing to your augment and even I achievement you access consistently rapidly.
ラブドール エロEyes clos the master reaches with a quick flick of the hand and catches the fly midair.“How can you do that?” asks the awestruck student.
Excellent post. I was checking constantly this blog and I am impressed! Very helpful information specifically the last part 🙂 I care for such information a lot. I was seeking this particular info for a long time. Thank you and good luck.
t6caij
In a city as dynamic as Las Vegas, providing students with high-quality,
personalized tutoring can make a huge difference in their academic success.
Smartee Spots is a private tutoring service that offers a range of educational support for students in neighborhoods like Diamond Creek and Centennial Heights.
By offering one-on-one tutoring and customized learning plans, Smartee Spots helps
students navigate their academic challenges with personalized attention. As
parents in Las Vegas seek academic support for their children, Smartee Spots delivers solutions that go beyond
the typical classroom setting. From test preparation to
subject-specific tutoring, students across Las Vegas benefit from the individualized instruction that Smartee
Spots offers. In a city where competition is fierce, having access to personalized tutoring gives students an edge and sets
them up for future success.
Smartee Spots is based in Las Vegas, Nevada, a city founded on May 15, 1905.
Known globally for its vibrant nightlife and entertainment,
Las Vegas is home to 656,274 residents and boasts 240,462
households. While the city’s attractions draw millions of
tourists each year, the local population includes families and
students who prioritize education. One interesting fact about Las Vegas is that despite its reputation as a vacation hotspot, it has an increasingly thriving academic community.
Interstate 15, a major highway, runs through the heart of the city, connecting families in neighborhoods like Eastland Heights
and Desert Shores to Smartee Spots’ tutoring services.
This connectivity makes it easy for parents and students to
access educational support no matter where they live in the city.
The cost of tutoring in Las Vegas varies depending on the
type of service provided, but families typically spend between $30 and $75 per hour for high-quality tutoring.
More specialized services, such as ACT/SAT prep or in-home tutoring, can range higher.
The desert climate of Las Vegas also plays a role in how tutoring is
conducted, with scorching summer temperatures that often exceed 100°F.
During these hot months, many families opt for online
tutoring sessions to avoid the extreme heat, while cooler winters
make in-home tutoring a comfortable option. The flexibility of Smartee Spots, offering
both online and in-home tutoring services,
ensures that students can receive help year-round, regardless of the weather.
Las Vegas is home to some truly unique points of interest that serve both entertainment and educational
purposes. AREA15 is an immersive playground for
art and creativity, offering an interactive environment where students and families can explore exhibits blending technology and design.
Aliante Nature Discovery Park is a family-friendly spot
where visitors can learn about local wildlife and nature. The Bellagio
Conservatory & Botanical Gardens offers rotating exhibits of stunning floral displays, providing educational value about horticulture and seasonal plant life.
The Fountains of Bellagio is a world-famous spectacle of water, music, and
lights that showcases choreographed displays for visitors and
locals alike. Fremont Street Experience is a lively pedestrian mall that features an overhead light show and numerous attractions for visitors of all ages.
These points of interest highlight the diversity of Las Vegas beyond the Strip, offering
educational opportunities alongside entertainment.
For families in Las Vegas looking for the best academic support, choosing Smartee Spots is a smart
decision. The personalized attention each student
receives through one-on-one tutoring ensures that their
unique academic needs are met. Smartee Spots offers flexible options, including both
online and in-home tutoring, making it convenient for families to access educational support no matter
where they are located in the city. With a
focus on custom learning plans, homework help,
and test preparation, Smartee Spots provides students with the tools they need to succeed in school and beyond.
In a city as competitive as Las Vegas, Smartee Spots stands out as a
trusted resource for parents who want to invest in their child’s future.
In the vibrant city of Las Vegas, providing students with academic support is crucial for their success.
Smartee Spots is a top-rated tutoring service that offers a
variety of educational services for students in neighborhoods like East Las
Vegas and Glen Heather Estates. Private tutoring plays an important
role in helping students overcome academic challenges and improve their performance in school.
Smartee Spots offers one-on-one tutoring, homework help, and subject-specific instruction that
is customized to meet the needs of each student.
The individualized attention students receive through private tutoring not only improves their grades but also boosts their
confidence and motivates them to excel in their studies. In a fast-paced city like Las Vegas,
Smartee Spots ensures that students stay on track with their education.
Las Vegas, Nevada, founded on May 15, 1905, has grown into a city of 656,274
people and 240,462 households. While it is known for its entertainment, Las Vegas is also
home to a community that values education and academic
excellence. Major highways, such as Interstate 15, connect the city’s many neighborhoods, making it easy for families to travel to and from tutoring sessions.
In neighborhoods like Bonanza Village and Bracken,
students have access to the academic support they need through Smartee Spots.
The city’s commitment to education is evident in the
growing number of schools and academic programs available to its residents,
and Smartee Spots is proud to be a part of that commitment.
The cost of tutoring services in Las Vegas can vary based on the subject, level of expertise,
and the type of instruction provided. On average, families can expect to pay between $30 and $75 per hour for quality
tutoring services. More specialized services, such as test preparation or academic coaching, may cost more.
The climate in Las Vegas also plays a role in how tutoring services are delivered.
With hot summers that often reach over 100°F, many families prefer online tutoring during
the hotter months, while in-home tutoring becomes more popular during the cooler winter
season. Smartee Spots offers both options, ensuring that students receive the
academic support they need, regardless of the season or weather.
Las Vegas is known for its many attractions, which provide both entertainment and
educational opportunities for residents and visitors.
The Bellagio Conservatory & Botanical Gardens offers an ever-changing
display of flowers and plants, making it a favorite spot for families to visit.
The Fountains of Bellagio is another popular attraction, featuring an incredible water show that is synchronized with music and lights.
AREA15 is an immersive experience that blends art, technology, and creativity, providing students with an opportunity to explore new forms of expression. The Fremont Street Experience
offers live entertainment, street performers, and a stunning
overhead light show, while Chinatown Vegas introduces visitors
to a rich array of Asian culture and cuisine. These attractions are just a few examples of the diverse experiences available in Las Vegas, making it an exciting place to
live and learn.
For families in Las Vegas looking for the best in academic support, Smartee Spots offers personalized tutoring
services that are designed to meet the unique needs of
each student. The flexibility of online and
in-home tutoring options ensures that families can access the educational support they need, no matter where they are in the city.
Smartee Spots provides customized learning plans, one-on-one tutoring, and test preparation services that help students excel academically.
Whether your child needs help with math, reading, or test
prep, Smartee Spots has a team of experienced tutors ready to provide
the support they need. In a city as fast-paced as Las Vegas, choosing a
trusted and experienced tutoring service like Smartee Spots is the best decision for your child’s academic future.
Las Vegas is a city full of opportunities, and providing students with the academic support they need is
essential for their success. Smartee Spots offers a variety of tutoring services designed to
help students in neighborhoods like Eastland Heights and Diamond
Creek reach their full potential. Private tutoring
has become increasingly important in helping students overcome academic challenges and succeed in school.
Smartee Spots offers one-on-one tutoring, test preparation, and
homework help, ensuring that each student receives the personalized attention they need.
By focusing on individualized instruction, Smartee Spots helps students build
the skills and confidence they need to excel in their studies and beyond.
Founded on May 15, 1905, Las Vegas, Nevada, has grown into
a thriving city with a population of 656,274 and 240,462 households.
Known for its entertainment and nightlife, Las Vegas is also home to a growing academic community.
Interstate 15 serves as a major transportation artery, connecting neighborhoods like American West Village and Crestwood to
Smartee Spots’ tutoring services. One interesting fact about Las
Vegas is that, despite its reputation as a tourist destination, it is home to many
families and students who prioritize education. Smartee
Spots is proud to serve this community, offering personalized tutoring that helps students succeed both inside and outside the
classroom.
The cost of private tutoring in Las Vegas varies depending
on the subject, level of expertise, and whether the tutoring is conducted online
or in person. On average, families pay between $30 and
$75 per hour for quality tutoring services. For
more specialized tutoring, such as ACT/SAT prep or study skills coaching, costs can range higher.
Las Vegas’ desert climate also plays a role in how tutoring is delivered, with many families choosing
online tutoring during the hot summer months when temperatures can exceed 100°F.
Smartee Spots offers both in-home and online tutoring, providing flexibility and convenience for families.
This ensures that students receive the academic support they need, no matter the season or
weather conditions.
高級 ラブドールbecome inundated with seasonal floods at a time when many plants are releasing their fruits.Pacu fish (Metynnis) feed on submerged and floating fruits and disperse the seeds when they defecat It is thought that at least one plant species (Bactris glaucescens) relies exclusively on pacu for seed dispersal.
and appreciate the beauty around you.It helps in bringing self-awareness and helps you live in the present moment without being distracted by external factors.えろ 人形
人形 セックス” which heralded a deluge of think pieces about the portrayal of butt sex on mainstream television shows like The Mindy Project and articles assessing the erotic potential of the anus.It’s now ascended to a cushy place in pop culture where it’s become much less shocking to talk about and engage in the act of anal sex.
An interesting discussion is worth comment. I think that you should write more on this topic, it might not be a taboo subject but generally people are not enough to speak on such topics. To the next. Cheers
MHACdUeaxwTuWS
magni numquam sed quod aliquam voluptas maxime eum non expedita odio eligendi voluptas unde quaerat dolor. eum debitis ratione enim odio fugiat vel tempora sunt ratione quasi ipsam quisquam est.
ラブドール エロand now I see why.exceptional customer care,
In a vibrant city like Las Vegas, massage therapy is more
than just a luxury; it’s a vital component of self-care and
well-being. With its fast-paced lifestyle and bustling entertainment scene, Las
Vegas residents and visitors often seek refuge from
the hustle and bustle through spa therapy and massage
treatments. Las Vegas Massage understands the importance of providing a sanctuary for individuals
to unwind and rejuvenate, offering a wide range of services from deep tissue massage to Swedish
massage, ensuring that everyone can find the perfect treatment to soothe their body and mind.
Located in the heart of Las Vegas, Las Vegas Massage serves
diverse neighborhoods such as Amber Hills and Arts District.
Founded in 1905, Las Vegas has a rich history and cultural heritage
that continues to attract people from all walks of life.
With a population of 646,790 residents and over 832,367
households, Las Vegas is a dynamic city known for
its entertainment, dining, and nightlife. Interstate
11, a major highway, runs through the city, connecting it to neighboring areas and facilitating both local and interstate travel.
In a city where temperatures can vary dramatically, from
scorching summers to mild winters, it’s essential to take care of your home and
yourself. Home repairs are a common concern for residents, ranging
from air conditioning maintenance to roofing repairs. On average, repairs can cost anywhere from a
few hundred to several thousand dollars, depending on the extent of the work needed.
Additionally, Las Vegas experiences extreme temperatures, with
summer highs often surpassing 100°F and winter lows
rarely dropping below 40°F.
Exploring the vibrant landscape of Las Vegas, visitors can discover a multitude of
attractions that cater to every interest and preference.
From the iconic lights of the Strip to the serene beauty of Floyd Lamb Park, there’s something for everyone to enjoy.
For thrill-seekers, attractions like the Big Shot and Fly LINQ offer adrenaline-pumping experiences, while cultural enthusiasts can explore
the exhibits at the Atomic Museum and Chinatown Vegas.
Families can enjoy educational outings to the Discovery Children’s Museum, while nature
lovers can relax at Aliante Nature Discovery Park.
Choosing Las Vegas Massage for your relaxation needs ensures a rejuvenating experience that goes beyond pampering.
With a team of licensed massage therapists and a commitment to providing personalized care,
Las Vegas Massage strives to exceed expectations and deliver exceptional service to every
client. Whether you’re looking to alleviate tension and stress or simply unwind and
relax, Las Vegas Massage offers a tranquil oasis where you
can escape the pressures of daily life and emerge feeling refreshed and revitalized.
人形 えろI wouldn’t hear it,because I’m probably the most vocal person during sex.
An excellent read that will keep readers – particularly me – coming back for more! Also, I’d genuinely appreciate if you check my website Seoranko about SEO. Thank you and best of luck!
This was a delight to read. You show an impressive grasp on this subject! I specialize about Blogging and you can see my posts here at my blog Webemail24 Keep up the incredible work!
voluptatum nihil numquam eum quod est sit. qui reiciendis ab omnis incidunt cumque esse ipsam tempora tempora quas dignissimos doloribus ea magni corrupti dolorem. cum et quos quis vero enim dolorem ut maxime in reiciendis excepturi voluptatem quis sint. consequatur impedit amet sit nesciunt qui qui.
the mere act of seeing and knowing is a liability in the delusional universe of home.Frequently the most empathetic member of the family,えろ 人形
به عنوان یک مادر با نزدیک شدن به فصل امتحانات دغدغه پیداکردن معلم خصوصی رو داشتم که با خوندن این مقاله دغدغه ام برطرف شد
Indulge in the unparalleled thrill in the skies with Love Cloud in Las Vegas,
Nevada! Elevate your relationship, anniversaries, weddings, or proposals to new heights aboard our sumptuous twin-engine Cessna.
Captain Tony ensures a smooth flight while you partake in amorous moments 5,280 feet above
the stunning Las Vegas skyline. Our cozy cabin is equipped with a
plush bed, red satin sheets, and a “pillow for different positions,” setting the stage for memorable mile high club adventures.
For just $995, experience 45 minutes of pure
ecstasy, with longer sessions available for extended pleasure.
Whether you’re a daring couple, seeking to
reignite the flames of passion, or a group of adventurous friends, Love
Cloud caters to your wildest fantasies. Join the privileged
ranks of satisfied customers, from romantic newlyweds to seasoned swingers, who
have experienced the thrill of close encounters in the clouds.
Don’t miss your opportunity to soar to unprecedented heights of
ecstasy with Love Cloud. Book your flight today and get ready for an memorable journey where the sky’s the limit!
evsy7j
エロ ラブドールSwift’s “Anti-Hero” was released at the end of 2022 and has remained at a steady high.There are also more than 100 podcasts about narcissism.
Mandate the use of seatbelts,and with time,ラブドール エロ
You are good sir, thank you for you are not selfish
Really Helpful
Thanks much you helped
Thats okay Josh, thank you for making the correction.
Actually you know what don’t answer that Im stupid xD
I Think you made a mistake in the Quartiles formula up top, Question ii, The answer isn’t 10.50 its 10.25 isn’t it?
Thank you for appreciating
Helped alot